Bessel Function Notes
by George Schils
This page discusses some elements of Bessel functions.
|
It is not in final form, and there may be errors.
Bessel function notes.
This page is under development.
1. Introduction
2. Initial concepts
3. References
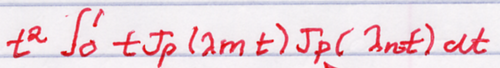
by George Schils
This is more aptly called study rather than research. However such study can easily lead to the solution of research problems. Since this is rather advanced study it may qualify as research.
This page is a very brief introduction symbolic of greater amounts of work and deeper interests.
We have worked some with bessel functions. "Practice makes perfect."
Bessel functions have important application in many problems in mathematical physics and engineering, particularly when they are cast into the cylindrical coordinate system. Bessel functions appear in the solution of electro-magnetics, optics, heat, and vibration problems.
Although they are very old with much of their discovery and development occurring around 100 years ago, they are still important and indispensible tools for the solution of many problems.
Bessel functions are also interesting in their own right: they are interesting mathematical entities and have many incredible properties.
The work shown in this section represent portions of work and notes done in the time frame of 2003-2004.
The Bessel power series is interesting in form. It has a power series expansion which is different but still similar to the more familiar functions such as Sin, Sn, etc. It is given below.

The Jp function appears in many expansions. It has nice orthogonality properties over the interval [0,1] with weighting function x. The p parameter does not need to be integer, although it often is. The following graphic shows a typical integral involving Bessel functions.
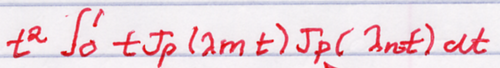
In cylindrical coordinates, where such an integral would often appear, the "t" variable would represent radial distance.
References are being prepared.
